Oscillation MCQs For NEET 2023

Oscillations

Question 1. The displacement of a particle executing simple harmonic motion is given by, y=\(y=A_0+A \sin \omega t+B \cos \omega t\) Then the amplitude of its oscillation is given by :

1. \(\sqrt{A^2+B^2}\)
2. \(\sqrt{A_0^2+(A+B)^2}\)
3. A+B
4. \(A_0+\sqrt{A^2+B^2}\)

Answer: 1. \(\sqrt{A^2+B^2}\)

Here: y=\(\mathrm{A}_{\mathrm{o}}+A \sin \omega t+B \cos \omega t\)

Equating with SHM ,\( y^{\prime} =y-\mathrm{A}_{\mathrm{o}}=\mathrm{A} \cos \left(\frac{\pi}{2}-\omega t\right)+\mathrm{B} \cos \omega \mathrm{t}\)

=A \(\sin \omega t+B \cos \omega \mathrm{t}\) .

Resultant amplitude, R =\(\sqrt{A^2+B^2+2 A B \cos 90^{\circ}}\)

=\(\sqrt{A^2+B^2}\)

Question 2. The distance covered by a particle undergoing SHM in one time period is (amplitude = A):

1. zero
2. A
3. 2A
4. 4A

Answer: 4. 4A

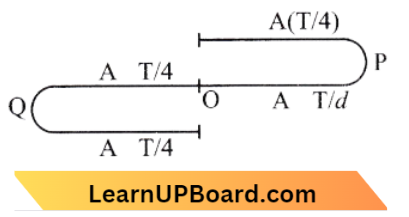
In an SHM particle moves from the mean position to an extreme position (P) and then returns to the mean position covering the same distance A.

A + A + A + A = 4 A

Question 3. When two displacement represented by \(y_1=a \sin (\omega t)\) and \(y_2=b \cos (\omega t)\) are superimposed, the motion is:

1. not a simple harmonic
2. simple harmonic with amplitude
3. simple harmonic with amplitude \(\sqrt{a^2+b^2}\)
4. simple harmonic with amplitude \(\frac{(a+b)}{2}\)

Answer: 3. simple harmonic with amplitude \(\sqrt{a^2+b^2}\)

From the question, \(y_1 =a \sin \omega t\)

⇒ \(y_2=b \cos \omega t \)

=b \(\sin \left(\omega t+\frac{\pi}{2}\right)\)

Read and Learn More NEET Physics MCQs

Resultant displacement is y=\(y_1+y_2\)=a \(\sin \omega t+b \sin \left(\omega t+\frac{\pi}{2}\right)\)

{Since \(\sin (A+B)\)=\(\sin A \cos B+\cos A \sin B\}\)

y=a \(\sin \omega t+b \sin (\omega t) \cos \frac{\pi}{2}+b \cos (\omega t) \sin \left(\frac{\pi}{2}\right)\)

y= \(a \sin (\omega t)+b \cos (\omega t)\)

Again Let b \(\cos (\omega t)=\mathrm{A} \cos \theta\) →  Equation 1

and a \(\sin (\omega t)=\mathrm{A} \sin \theta\)  → Equation 2

Squaring and Adding eq. (1) and (2)

A = \(\sqrt{a^2+b^2}\)

y = \(\sqrt{a^2+b^2} \sin (\omega t+\theta)\)

∴ \(\sqrt{a^2+b^2}\) is the amplitude of SHM

Question 4. Out of the following functions representing motion of a particle which represents SHM:

1. y=\(\sin \omega t-\cos \omega t\)
2. y=\(\sin ^3 \omega t\)
3. y=\(5 \cos \left(\frac{3 \pi}{4}-3 \omega t\right)\)
4. y=\(1+\omega t+\omega^2 t^2\)

1. Only (4) does not represent SHM
2. (1) and (3)
3. (1) and (2)
4. Only (1)

Answer: 3. (1) and (2)

For a S.H.M. \(\mathrm{a} \alpha \frac{d^2 y}{d t^2} \alpha-y\)

Hence equation y=\(\sin \omega t-\cos \omega t\) and

y= \(5 \cos \left(\frac{3 \pi}{4}-3 \omega t\right)\) are satisfying this condition.

Question 5. Two particles are oscillating along two close parallel straight lines side by side, with the same frequency and amplitudes. They pass each other, moving in opposite directions when their displacement is half of the amplitude. The mean positions of the two particles lie in a straight line perpendicular to the paths of the two particles. The phase difference is:

1. zero
2. \(\frac{2 \pi}{3}\)
3. \(\pi\)
4. \(\frac{\pi}{6}\)

Answer: 2. \(\frac{2 \pi}{3}\)

The Equation becomes, \(y_1 =\frac{A}{2}=A \sin \omega t \)

⇒ \(\omega t =30^{\circ}\)

⇒ \(y_2 =\frac{A}{2}=A \sin \left(\omega t+\frac{\pi}{2}\right)\)

= \(\omega t+\frac{\pi}{2}=150^{\circ}\)

phase difference \(\Delta \phi =\phi_2-\phi_1 \)

= \(150-30=120^{\circ}\)

= \(\frac{2 \pi}{3} \mathrm{rad}\)

Question 6. The displacement of a particle along the x-axis is given by x = \(x=\mathrm{a} \sin ^2 \omega t\). The motion of the particle corresponds to:

1. simple harmonic motion of frequency \(\omega / \pi\)
2. simple harmonic motion of frequency\(3 \omega / 2 \pi\)
3. nonsimple harmonic motion
4. simple harmonic motion of frequency \(\pi\omega / 2 \)

Answer: 3. nonsimple harmonic motion

According to the question, x=a \(\sin ^2 \omega t\) →  Equation 1

Differentiating eq. (1) w.r.t. t we get

⇒ \(\frac{d x}{d t}=2 a \omega(\sin \omega t)(\cos \omega t)\)

Again differentiating w.r.t. t we have

a=\(\frac{d^2 x}{d t^2} =2 a \omega^2\left[\cos ^2 \omega t-\sin ^2 \omega t\right]\)

=2 a \(\omega^2 \cos 2 \omega t\)  → Equation 2

The eq. (2) does not satisfy the condition of SHM’ So, motion is not simPle harmonic’

Question 7. A particle executes simple harmonic oscillation with an amplitude a. The period of oscillation is T. The minimum time taken by the particle to travel half of the amplitude from the equilibrium position is

1. \(\frac{T}{8}\)
2. \(\frac{T}{12}\)
3. \(\frac{T}{2}\)
4. \(\frac{T}{4}\)

Answer: 2. \(\frac{T}{12}\)

For equilibrium position, x(t)=a \(\sin \omega t\)

At, x(t)=\(\frac{a}{2}\)

⇒ \(\sin(\frac{\pi}{6})=\sin \omega t {\omega=\frac{2 \pi}{\mathrm{T}}}\)

or \(\frac{\pi}{6}=\frac{2 \pi t}{T}\)

t=\(\frac{T}{12}\)

Question 8. The circular motion of a particle with constant speed is:

1. periodic but not simple harmonic
2. simple harmonic but not periodic
3. period and simple harmonic
4. neither periodic nor simple harmonic

Answer: 1. periodic but not simple harmonic

A particle is a constant-speed circular motion that repeats its motion at regular intervals but does not oscillate around a fixed location. As a result, particle motion is periodic but not simply harmonic’

Question 9. Two simple harmonic motions with the same frequency act on a particle at right angles i.e. along X-axis and 7-axis. If the two amplitudes are equal and the phase difference is \(\frac{\pi}{2}\) the resultant motion will be:

1. a circle.
2. an ellipse with the major axis along the Y-axis.
3. an ellipse with the major axis along the X-axis.
4. a straight line inclined at 45° to the X-axis.

Answer: 1. a circle

The equations of two simple harmonic motions can be written as x=\(a \sin \omega t \) Equation 1

and y=\(a \sin \left(\omega t+\frac{\pi}{2}\right)\)

y=\(a \cos \omega t\) Equation 2

On squaring and adding Eqs. (1) and (2), we get

⇒ \(x^2+y^2 =a^2\left(\sin ^2 \omega t+\cos ^2 \omega t\right)\)

or \(x^2+y^2 =a^2\)

It represents the equation of a circular motion with radius a.

Question 10. A Simple harmonic oscillator has an amplitude of a and a period of T. The time required by it to travel from,\(x=a \text { to } x=\frac{a}{2}\) is:

1. \(\frac{T}{6}\)
2. \(\frac{T}{4}\)
3. \(\frac{T}{3}\)
4. \(\frac{T}{2}\)

Answer: 1. \(\frac{T}{6}\)

We have, the equation of simple harmonic motion given by,

or x =\(a \sin \omega t\)

= \(a \sin \left(\frac{2 \pi}{T}\right) t\)

when, x =a , then

a = \(a \sin \left(\frac{2 \pi}{T}\right) t \)

⇒ \(\sin \left(\frac{2 \pi}{T}\right) t\) =1

⇒ \(\sin \left(\frac{2 \pi}{T}\right) t =\sin \frac{\pi}{2}\)

t =\(\frac{T}{4}\)

when x=\(\frac{a}{2}\), then

⇒ \(\frac{a}{2} =a \sin \left(\frac{2 \pi}{T} \cdot t\right)\)

⇒ \(\sin \left(\frac{2 \pi}{T} t\right) =\sin \frac{\pi}{6}\)

t=\(\frac{T}{12}\)

Hence, the time taken to travel from

x=a to x=\(\frac{a}{2}=\frac{T}{4}-\frac{T}{12}=\frac{T}{6}\)

Question 11. The composition of two simple harmonic motions of equal periods at a right angle to each other and with a phase difference of \(\pi\) results in the displacement of the particles along:

1. circle
2. figure of eight
3. straight line
4. ellipse

Answer: 3. straight line

For simple harmonic motion,

x =\(a \sin \omega \mathrm{t}\)  →  Equation 1

and y =\(b \sin (\omega \mathrm{t}+\pi)\)

y =\(-b \sin \omega \mathrm{t}\)  →  Equation 2

or From eq. (1) and (2), we get

⇒ \(\frac{x}{a} =\sin \omega \mathrm{t} \)

and \(-\frac{y}{b} =\sin \omega \mathrm{t}\)

⇒ \(\frac{x}{a} =-\frac{y}{b}\)

y =\(-\frac{b}{a} x\)

Hence, it is an equation of a straight line

Question 12. The radius of the circle, the period of revolution, the initial position, and the sense of revolution are indicated in the below figure. y-projection of the radius vector of rotating particle P is :

1. y(t)=\(4 \sin \left(\frac{\pi t}{2}\right)\), where y in m
2. y(t)=\(3 \cos \left(\frac{3 \pi t}{2}\right)\), where y in m
3. y(t)=\(3 \cos \left(\frac{\pi t}{2}\right)\), where y in m
4. y(t)=\(-3 \cos 2 \pi t\), where y in m

Answer: 3. y(t)=\(3 \cos \left(\frac{\pi t}{2}\right)\), where y in m

Here, T=4 s, A=3 m

Time Period, \(\mathrm{T}=\frac{2 \pi}{\omega}\)

⇒ \(\omega =\frac{2 \pi}{T}=\frac{2 \pi}{4}=\frac{\pi}{2} \mathrm{rad} / \mathrm{s}\)

y =a cost \(\omega t=3 \cos \frac{\pi}{2} t\)

Question 13. A particle executes linear simple harmonic motion with an amplitude of 3 cm. When the particle is at 2 cm from the mean position, the magnitude of the velocity is equal to that of its acceleration. Then, its period in seconds is

1. \(\frac{\sqrt{5}}{\pi}\)
2. \(\frac{\sqrt{5}}{2 \pi}\)
3. \(\frac{4 \pi}{\sqrt{5}}\)
4. \(\frac{2 \pi}{3}\)

Answer: 3. \(\frac{4 \pi}{\sqrt{5}}\)

Given the magnitude of the velocity of a particle = magnitude of the acceleration of a particle in SHM  →  Equation 1

The magnitude of the velocity of grice at a displacement from the mean position is, \(\omega \sqrt{A^2-y^2}\), and the magnitude of the acceleration of particles in SHM is \(\omega^2 y\)

Then eq. (1) becomes,

⇒ \(\omega \sqrt{A^2-y^2} =\omega^2 y \)

⇒ \(\omega =\frac{\sqrt{A^2-y^2}}{y}=\frac{\sqrt{(3)^2-(2)^2}}{2}\)

= \(\frac{\sqrt{9-4}}{2}=\frac{\sqrt{5}}{2}\)

Time period, T=\(\frac{2 \pi}{\omega}=\frac{2 \pi}{\sqrt{5} / 2}=\frac{4 \pi}{\sqrt{5}}\)

Time period, T=\(\frac{4 \pi}{\sqrt{5}} s\)

Question 14. Two simple harmonic motions given by, \(x=a \sin \omega \mathrm{t}+\lambda \text { and } y=a \sin \left(\omega t+\delta+\frac{\pi}{2}\right)\)act on a particle simultaneously, then the motion of particle will be

1. circular anti-clockwise
2. circular clockwise
3. elliptical anti-clockwise
4. elliptical clockwise

Answer: 2. circular clockwise

x= a \(\sin (\omega t+\delta)\)

y= \(a \sin \left(\omega t+\delta+\frac{\pi}{2}\right)\)

or y= a \(\cos (\omega t+\delta)\)

Squaring and adding eq. (i) and (ii), we get

⇒ \(x^2+y^2=a^2\left[\sin ^2(\omega t+\delta)+\cos ^2(\omega t+\delta)\right]\)

or \(x^2+y^2=a^2\left(\sin ^2 \theta+\cos ^2 \theta=1\right)\)

This represents a circle.

At \((\omega t+\delta)\)=0 ; x=0, y=a

At \((\omega t+\delta)=\frac{\pi}{2}\) ; x=a, y=0

At \((\omega t+\delta)=\pi\) ; x=0, y=-a

At\( (\omega t+\delta)=\frac{3 \pi}{2}\) ; x=-a, y=0

At \((\omega t+\delta)=2 \pi\) ; x=0, y=a

It is clear That the particle motion is in a circular clockwise direction.

Question 15. The phase difference between displacement and acceleration of a particle in a simple harmonic motion is :

1. \(\frac{3 \pi}{2} \mathrm{rad}\)
2. \(\frac{\pi}{2} \mathrm{rad}\)
3. zero
4. \(\pi \mathrm{rad}\)

Answer: 4. \(\pi \mathrm{rad}\)

In Simple Harmonic Motion, The equation of displacement of the particle is y=\(a \sin \omega t\) → Equation 1

And the equation of acceleration of the particle is, a \(\omega^2 \sin \omega t=a \omega^2 \sin (\omega t+\pi)\)  → Equation 2

From eq. (1) and equation (2)

The phase difference between displacement and acceleration of the particle is :

⇒ \(\phi=(\omega t+\pi)-\omega t\)

⇒ \(\phi=\omega t+\pi-\omega t=\pi \text { radian }=\pi \mathrm{rad}\) .

Question 16. The average velocity of a particle executing SHM complete vibration is :

1. \(A \omega\)
2. \(\frac{A \omega^2}{2}\)
3. zero
4. \(\frac{A \omega}{2}\)

Answer: 3. zero

In one complete vibration, displacement is \(2 \pi \omega\). So, the average velocity in one complete vibration is :

∴ \(\frac{\text { Displacement }}{\text { Time Interval }}=\frac{y_1-y_2}{\mathrm{~T}}\)=0

Question 17. The particle is executing SHM along a straight line. Its velocities at distance x₁ and x₂ from the mean position or v₁ and v₂ respectively. Its period is:

1. \(2 \pi \sqrt{\frac{x_1^2+x_2^2}{v_1^2+v_2^2}}\)
2. \(2 \pi \sqrt{\frac{x_1^2-x_2^2}{v_1^2-v_2^2}}\)
3. \(2 \pi \sqrt{\frac{v_1^2+v_2^2}{x_1^2+x_2^2}}\)
4. \(2 \pi \sqrt{\frac{v_1^2-v_2^2}{x_1^2-x_2^2}}\)

Answer: 2. \(2 \pi \sqrt{\frac{x_1^2-x_2^2}{v_1^2-v_2^2}}\)

We know under SHM if A is the amplitude of  oscillation, then the velocity

⇒ \(v_1^2=\omega^2\left(\mathrm{~A}^2-x_1^2\right)\)

⇒ \(v_2^2=\omega^2\left(\mathrm{~A}^2-x_2^2\right)\)

Where \(x_1\) and \(x_2\) are the displacement of particles from the mean position,

Subtracting eq. (2) from eq (1) we have,

⇒ \(v_2^2-v_1^2 =\omega^2\left(x_2^2-x_1^2\right)\)

⇒ \(\omega =\sqrt{\frac{v_2^2-v_1^2}{x_2^2-x_1^2}}\)

⇒ \(\frac{2 \pi}{T}=\sqrt{\frac{v_2^2-v_1^2}{x_2^2-x_1^2}}\)

T =\(2 \pi \sqrt{\frac{x_2^2-x_1^2}{v_2^2-v_1^2}}\)

Question 18. A particle is executing a simple harmonic motion. Its maximum acceleration is \(\alpha\) and its maximum velocity is \(\beta\). Then, its period of vibration will be:

1. \(\frac{\beta^2}{\alpha^2}\)
2. \(\frac{\alpha}{\beta}\)
3. \(\frac{\beta^2}{\alpha}\)
4. \(\frac{2 \pi \beta}{\alpha}\)

Answer: 4. \(\frac{2 \pi \beta}{\alpha}\)

Acceleration of particles does.ng SHM is,

⇒ \(\alpha=A \omega^2\)  → Equation 1

A= maximum amplitude

⇒ \(\omega\)= angular velocity of particle

Again Maximum velocity,

⇒ \(\beta=A \omega\)  → Equation 2

From eq. (1) and (2) we have

⇒ \(\frac{\alpha}{\beta}=\frac{A \omega^2}{A \omega}=\omega=\frac{2 \pi}{T}\)

T=\(\frac{2 \pi \beta}{\alpha}\)

Time period of vibration, T=\(\frac{2 \pi \beta}{\alpha}\)

Question 19. The oscillation of a body on a smooth horizontal surface is represented by the equation, x = \(x=A \cos (\omega t)\)

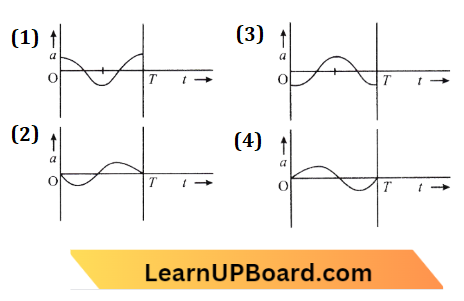
1. where, x = displacement at time t
2. \(\omega\) = frequency of oscillation
3. Which one of the following graphs shows correctly the variation a with t?
4. Here, a = acceleration at time t,T= time period

Answer: 1. where, x = displacement at time t

The oscillation of the body on a smooth horizontal surface u.

X= \(A \cos \omega t\)

Where, X= displacement at t

⇒ \(\omega\)= frequency of oscillation

A=Amplitude

Here at t=0, X=A

at t=\(\frac{T}{4}, X=A \cos \left(\frac{2 \pi}{T} \times \frac{T}{4}\right)=A \cos \frac{\pi}{2}\)=0

at \(t=\frac{T}{2}, X=A \cos \left(\frac{2 \pi}{T} \times \frac{T}{2}\right)=\mathrm{A} \cos \pi=-\mathrm{A}\)

Question 20. A particle of mass m oscillates along the x-axis according to equation x = a sin \(\omega\). The nature of the graph between the momentum and displacement of the particle is:

1. Circle
2. Hyperbola
3. Ellipse
4. A straight line passing through the origin

Answer: 3. Ellipse

According to the question, x =\(\mathrm{a} \sin \omega t\)

⇒ \(\frac{x}{a} =\sin \omega t\)  → Equation 1

Now velocity v=\(\frac{d x}{d t}=a \omega \cos \omega t\)

⇒ \(\frac{v}{a \omega}=\cos \omega t\)  →  Equation 2

From eq. (1) and (2)

⇒ \(\frac{x^2}{a^2}+\frac{v^2}{a^2 \omega^2}=\sin ^2 \omega t+\cos ^2 \omega t\)

⇒ \(\frac{x^2}{a^2}+\frac{v^2}{a^2 \omega^2}\)=1

∴ This is the equation of an ellipse. So the graph between the moment and displacement of the particle is ellipse.

Question 21. A simple pendulum performs simple harmonic motion x = 0 with an amplitude a and period T. The speed of the pendulum at x =\(\frac{a}{2}\) will be:

1. \(\frac{\pi a \sqrt{3}}{2 T}\)
2. \(\frac{\pi a}{T}\)
3. \(\frac{3 \pi^2 a}{T}\)
4. \(\frac{\pi a \sqrt{3}}{T}\)

Answer: 4. \(\frac{\pi a \sqrt{3}}{T}\)

We know that, V =\(\frac{d y}{d t}=A \omega \cos \omega t\)

= \(A \omega \sqrt{1-\sin ^2 \omega t}\)

= \(A \omega \sqrt{A^2-y^2}\)

Here, y =\(\frac{a}{2}\)

V = \(\omega \sqrt{a^2-\frac{a^2}{4}}=\omega \sqrt{\frac{3 a^2}{4}}\)

= \(\frac{2 \pi}{T} \frac{a \sqrt{3}}{2}=\frac{\pi a \sqrt{3}}{T}\)

Question 22. Which of the following equations of motion represents simple harmonic motion?

1. Acceleration = \(-k_0 x+k_1 x^2\)
2. Acceleration = – k(x + a)
3. Acceleration = k(x + a)
4. Acceleration = kx

Answer: 2. Acceleration = – k(x + a)

Here acceleration a(\(\alpha\)-displacement)

A \(\propto-y\)

A = \(-\omega^2 y \)

A = \(-\frac{k}{m} y\)

A =-k y

And y =x+a

acceleration =-k(x+a)

Question 23. Two simple harmonic motions of angular frequency 100 and 1000 rad \(\mathrm{s}^{-1}\) have the same displacement amplitude. The ratio of their maximum acceleration is:

1. 1: 10
2. 1: 10²
3. 1: 10³
4. 1: 10⁴

Answer: 2. 1: 10²

Acceleration of SHM is, \(a_{\max } =-\omega^2 A\)

or \(\frac{\left(a_{\max }\right)_1}{\left(a_{\max }\right)_2} =\frac{\omega_1^2}{\omega_2^2}\)(A remains same )

or \(\frac{\left(a_{\max }\right)_1}{\left(a_{\max }\right)_2} =\frac{(100)^2}{(1000)^2}=\left(\frac{1}{10}\right)^2 \)

= \(1: 10^2\)

Question 24. A point performs simple harmonic oscillation of period T and the equation of motion is given by x = \(x=a \sin (\omega t+\pi / 6)\). After the elapse of what fraction of the period the velocity of the point will be equal to half of its maximum velocity?

1. \(\frac{T}{8}\)
2. \(\frac{T}{6}\)
3. \(frac{T}{3}\)
4. \(\frac{T}{12}\)

Answer: 4. \(\frac{T}{12}\)

The equation is, x=\(a \sin \left(\omega t+\frac{\pi}{6}\right)\)  → Equation 1

Differentiating we get, v=\(\frac{d x}{d t}=a \omega \cos \left(\omega t+\frac{\pi}{6}\right)\) (Now v=\(\frac{a \omega}{2})\)

⇒ \(\frac{a \omega}{2}=a \omega \cos \left(\omega t+\frac{\pi}{6}\right)\)

⇒ \(\frac{1}{2}=\cos \left(\omega t+\frac{\pi}{6}\right)\)

⇒ \(\cos \frac{\pi}{3} =\cos \left(\omega t+\frac{\pi}{6}\right)\)

⇒ \(\omega t+\frac{\pi}{6} =\frac{\pi}{3}\)

or \(\omega t =\frac{\pi}{6}\)

t =\(\frac{\pi}{6 \omega}=\frac{\pi \times T}{6 \times 2 \pi}=\frac{T}{12}\)

Thus at\( \frac{T}{12}\) velocity of the point will be equal to half of its maximum velocity.

Question 25. The phase difference between the instantaneous velocity and acceleration of a particle executing simple harmonic motion is :

1. \(\pi\)
2. \(0.70 \pi\)
3. zero
4. \(0.5 \pi\)

Answer: 4. \(0.5 \pi\)

Let y=\(A \sin \omega t=A \omega^2 \sin (\omega t+\pi)\)

⇒ \(\frac{d y}{d t} =A \omega \cos \omega t\)

= \(A \omega \sin \left(\omega t+\frac{\pi}{2}\right)\)

Acceleration,\(\frac{d^2 y}{d x^2}=-A \omega^2 \cos \omega t=A \omega^2 \sin (\omega t+\pi)\)

Phase difference =\(\pi-\frac{\pi}{2}\)

Question 26. A particle executing simple harmonic motion of amplitude 5 cm has a maximum speed of 31.4 cm/s. The frequency of its oscillation is:

1. 4 Hz
2. 3 Hz
3. 2 Hz
4. 1 Hz

Answer: 4. 1 Hz

Here a=5 \(\mathrm{~cm}, V_{\max }=\frac{31.4 \mathrm{~cm}}{\mathrm{~s}}\)

⇒ \(V_{\max }=\omega a=31.4=2 \pi \mathrm{v} \times 5\)

31.4=10 \(\times 3.14 \times v \)

v=1 \(\mathrm{~Hz}\)

Question 27. Which one of the following statements is true for the speed v and the acceleration a of a particle executing simple harmonic motion?

1. When v is maximum, a is maximum
2. The value of a is zero, whatever may be the value of v
3. When v is zero, a is zero
4. When v is maximum a is zero

Answer: 4. When v is maximum a is zero

In SHM, Velocity = \(A \omega \sin \left(\omega t+\frac{\pi}{2}\right)\)

Acceleration=A \(\omega^2 \sin (\omega t+\pi)\)

This shows that v is max then a is zero.

Question 28. A particle starts a simple harmonic motion from the mean position. Its amplitude is a and its period is T. What is its displacement when its speed is half of its maximum speed?

1. \(\frac{\sqrt{2}}{3} a\)
2. \(\frac{\sqrt{3}}{2} a\)
3. \(\frac{2}{\sqrt{3}} a\)
4. \(\frac{a}{\sqrt{2}} a\)

Answer: 2. \(\frac{\sqrt{3}}{2} a\)

At any given time, the velocity of the particle executing SHM is defined as the rate of change of its displacement.

Let the displacement of the particle at an instant be given by

Velocity, v = \(\frac{d x}{d t}=\frac{d(a \sin \omega t)}{d t}\)

=\(a \omega t \cos \omega t\)

= \(a \omega \sqrt{\left(1-\sin ^2 \omega t\right)}\)

= \(a \omega \sqrt{\left(1-\frac{x^2}{a^2}\right)}=w \sqrt{\left(a^2-x^2\right)}\)

At mean position, x=0

⇒ \(v_{\max }=\omega a\)

According to question, v=\(\frac{\mathrm{v}_{\max }}{2}=\frac{\mathrm{a} \omega}{2}\)

But, v=\(\omega \sqrt{a^2-x^2}\)

= \(\frac{a \omega}{2}=\omega \sqrt{a^2-x^2}\)

Or x=\(\frac{\sqrt{3}}{2} a\)

Question 29. A body executes SHM with an amplitude a. At what displacement from the mean position, the potential energy of the body is one-fourth of its total energy?

1. \(\frac{\mathrm{a}}{4}\)
2. \(\frac{a}{2}\)
3. \(\frac{3 a}{4}\)
4. Some other fraction of a

Answer: 2. \(\frac{a}{2}\)

We have, the potential energy of a body executing SHM, U=\(\frac{1}{2} m \omega^2 x^2\)

The total energy of the body executing SHM,

E=\(\frac{1}{2} m \omega^2 a^2\)

According to the question, U =\(\frac{1}{4} E \)

⇒ \(\frac{1}{2} m \omega^2 x^2 =\frac{1}{4} \times \frac{1}{2} m \omega^2 a^2 \)

⇒ \(x^2 =\frac{a^2}{4}\)

x =\(\frac{a}{2}\)

Question 30. A particle moving along the X-axis executes simple harmonic motion, and then the force acting on it is given by where A and K are positive constants.

1. – AKx
2. Acos Kx
3. Aexp(-Ax)
4. AKx

Answer: 1. – AKx

If a particle in simple harmonic motion moves x distant from its equilibrium position, the magnitude of the restoring force 17acting on the particle at that instant is given by F =-kx

where k is known as the force constant. Hence, in given options, option (A) is correct. Here, k = Ak.

Question 31. A body is executing simple harmonic motion with frequency V, the frequency of its potential energy is:

1. n
2. 2n
3. 3n
4. 4n

Answer: 1. n

Given, SHM frequency = n

PE frequency =?

Since PE frequency is the same as SHM frequency.

Question 32. A particle of mass m is released from rest and follows a parabolic path as shown. Assuming that the displacement of the mass from the origin is small, which graph correctly depicts the position of the particle as a function of time:

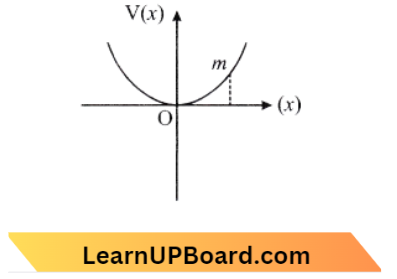
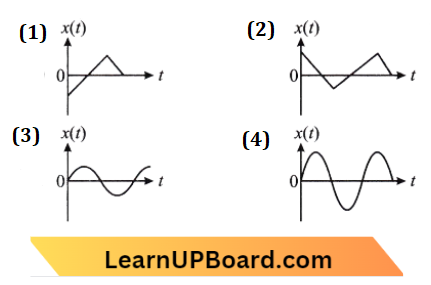
Answer: 4.

Motion starts from an extreme position and for small displacement it is SHM.

∴ \(y=\mathrm{A} \cos (\omega t+\phi)\)

Question 33. The particle executing simple harmonic motion has a kinetic energy \(K_0 \cos ^2 \omega t\)t. The maximum values of the potential energy and the total energy are respectively:

1. \(K_0 / 2\) and \( K_0\)
2. \(K_0 \)and \( 2 K_0\)
3. \(K_0 \)and \( K_0\)
4. \(0\) and \( 2 K_0\)

Answer: 3. \(K_0\) and \( K_0\)

⇒ \(K. E. =K_0 \cos ^2 \omega t\)

Maximum P. E. Maximum K. E. Total energy

= \(K_0\)

Question 34. A particle of mass m oscillates with simple harmonic motion between points X₁ and X₂, the equilibrium position being O. Its potential energy is plotted. It will be as given below in the graph:

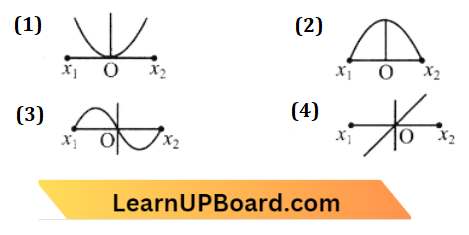
Answer: 1.

PE in SHM =\(\frac{1}{2} K x^2\) →  equation of parabola

Question 35. The potential energy of a simple harmonic oscillator when the particle is halfway to its endpoint is:

1. \(\frac{2}{3} E\)
2. \(\frac{1}{8} E\)
3. \(\frac{1}{4} E\)
4. \(\frac{1}{2} E\)

Answer: 3. \(\frac{1}{4} E\)

P.E. =\(\frac{1}{2} K x^2 \)

=\(\frac{1}{2} K\left(\frac{x}{2}\right)^2=\frac{E}{4}\)

Question 36. The displacement between the maximum potential energy (P.E.) position and the maximum energy (K.E.) position for a particle executing simple harmonic motion is:

1. \(\pm \frac{a}{2}\)
2. \(\pm \mathrm{a}\)
3. \(\pm 2 \mathrm{a}\)
4. \(\pm 1\)

Answer: 2. \(\pm \mathrm{a}\)

The K.E. of pendulum, K.E.=\(\frac{1}{2} K\left(a^2-y^2\right)\)

and P.E. of pendulum =\(\frac{1}{2} K Y^2\)

when y = 0 (mean position) K.E. is maximum and PE is 0

When: 0, K.E. is 0 and P.E. is maximum.

The displacement between the position of maximum potential energy and maximum kinetic energy is \(\pm\) a

Question 37. In SHM restoring force is F = – kx, where k is force constant, x is displacement and a is amplitude of motion, then total energy depends upon:

1. k, a and m
2. k, x, m
3. k, a
4. k, x

Answer: 3. k, a

In simple harmonic motion, the total energy = Potential energy + Kinetic energy

or E = U + K

= \(\frac{1}{2} m \omega^2 x^2+\frac{1}{2} m \omega^2\left(a^2-x^2\right)\)

= \(\frac{1}{2} m \omega^2 a^2+\frac{1}{2} k a^2 \)

Where, k = force constant = \(m \omega^2\)

Question 38. In a simple harmonic motion, when the displacement is one-half the amplitude, what fraction of the total energy is kinetic?

1. Zero
2. \(\frac{1}{4}\)
3. \(\frac{1}{2}\)
4. \(\frac{3}{4}\)

Answer: 4. \(\frac{3}{4}\)

The total energy of a particle executing SHM at instant time t,

E=\(\frac{1}{2} m^2 \omega a^2\)  → Equation 1

and kinetic energy of the particle at instant t,

⇒ \(E_K=\frac{1}{2} m \omega^2\left(a^2-x^2\right)\)  → Equation 2

when x =\(\frac{a}{2}\),

⇒  \(E_K =\frac{1}{2} m \omega^2\left(a^2-\frac{a^2}{4}\right)\)

=\(\frac{1}{2} m \omega^2 \times \frac{3}{4} a^2 \)

⇒ \(E_K =\frac{1}{2} \times \frac{3}{4} m \omega^2 a^2\) →  Equation 3

Or From Eqs. (1) and (3), we get

⇒  \(\frac{E_K}{E} =\frac{3}{4}\)

∴ \(E_K \frac{3}{4} E\)

Question 39. The angular velocity and the amplitude of a simple pendulum are co and a respectively. At a displacement x from the mean position, if its kinetic energy is T and potential energy is U, then the ratio of T to U is

1. \(\left(\frac{a^2-x^2 \omega^2}{x^2 \omega^2}\right)\)
2. \(\frac{x^2 \omega^2}{\left(a^2-x^2 \omega^2\right)}\)
3. \(\frac{\left(a^2-x^2\right)}{x^2}\)
4. \(\frac{x^2}{\left(a^2-x^2\right)}\)

Answer: 3. \(\frac{\left(a^2-x^2\right)}{x^2}\)

Let us consider a particle of mass m that performs linear SHM with an amplitude of, a and a constant angular frequency. Let us assume that the particle’s displacement is x, which is determined by r seconds after starting from the mean position.

We have, x=\(a \sin \omega t\)

So, the potential energy of the particle is, \(\mathrm{U}=\frac{1}{2} m \omega^2 x^2\) → Equation 1

and kinetic energy of particle is, T=\(\frac{1}{2} m \omega^2\left(a^2-x^2\right)\) →  Equation 2

From Eqs. (1) and (2), we get,

⇒  \(\frac{T}{U}=\frac{a^2-x^2}{x^2}\)

Question 40. A particle, with restoring force proportional to displace¬ment and resisting force proportional to velocity is subjected to a force F \(\sin \omega t\). If the amplitude of the particle is maximum for \(\omega=\omega_1\), and the energy of the particle is maximum for \(\omega=\omega_2\), then

1. \(\omega_1=\omega_0 and \omega_2 \neq \omega_0\)
2. \(\omega_1=\omega_0 and \omega_2=\omega_0\)
3. \(\omega_1 \neq \omega_0 and \omega_2=\omega_0 \)
4. \(\omega_1 \neq \omega_0 and \omega_2 \neq \omega_0\)

Answer: 3. \(\omega_1 \neq \omega_0 and \omega_2=\omega_0 \)

As we know the energy of the particle is maximum at natural frequency. Since the restoring force is proportional to the displacement and the resisting force is proportional to velocity.

So, \(\omega_1 \neq \omega_0 \)

And, \(\omega_2 =\omega_0\)

Question 41. Two pendulums of length 121 cm and 100 cm start vibrating in phase. At some instant, the two are at their mean position in the same phase. The minimum number of vibrations of the shorter pendulum after which the two are again in phase at the mean position is:

1. 11
2. 9
3. 10
4. 8

Answer: 1. 11

Let us consider,L=121 \(\mathrm{~cm}=1.21 \mathrm{~m} ; L^1=100 \mathrm{~cm}=1 \mathrm{~m}\)

T= longer pendulum; \(\mathrm{T}^1\)= shorter pendulum we know,

T=\(2 \pi \sqrt{\frac{l}{g}} \Rightarrow \mathrm{T} \propto \sqrt{l}\)

⇒  \(\frac{T}{T^1} \propto \sqrt{\frac{L}{L^1}}\)

⇒  \(\frac{T}{T^1} \propto \sqrt{\frac{1.21}{1}}=\frac{1.1}{1}\)

10 \(\mathrm{~T}=11 \mathrm{~T}^1\)

So, 10 vibrations of the longer pendulum: 1L vibration of a shorter pendulum

Question 42. A spring is stretched by 5 cm by a force of 10N. The period of the oscillations when a mass of 2 kg is suspended by it is:

1. 0.0628 s
2. 6.28 s
3. 3.14 s
4. 0.628 s

Answer: 4. 0.628 s

Given, Stretch(x)=5cm

Force (F) = 10 N

Mass (m) = 2ke

Time period, T =?

We know, that, the force constant of spring, \(\mathrm{K}=\frac{F}{x}\)

⇒  \(\mathrm{K} =\frac{10}{0.05}=200 \mathrm{~N} / \mathrm{m}\)

⇒  \(\omega =\frac{2 \pi}{\mathrm{T}}\)

⇒  \(\frac{4 \pi^2}{\mathrm{~T}^2} =\frac{k}{m}\)

⇒  \(\mathrm{~T}^2 =4 \pi^2 \frac{m}{k}\)

= \(-2 \pi \sqrt{\frac{m}{\mathrm{~K}}}\)

⇒  \(\mathrm{T}^2 =4 \pi^2 \frac{m}{k}\)

= \(-2 \pi \sqrt{\frac{m}{\mathrm{~K}}}\)

⇒  \(\mathrm{~T} =2 \pi \sqrt{\frac{2}{200}}\)

=\(2 \pi \sqrt{\frac{1}{100}}\)

=\(\frac{2 \pi}{10}\)

∴ \(\mathrm{~T} =\frac{6.28}{10}=0.628 \mathrm{~s}\)

Question 43. A pendulum is hung from the roof of a sufficiently high building and is moving freely from end to end like a simple harmonic oscillator. The acceleration of the bob of the pendulum is 20 m/s² at a distance of 5 m from the mean position. The period of oscillation is:

1. 2s
2. \(\pi s\)
3. 2 \(\pi s\)
4. 1 \(\mathrm{~s}\)

Answer: 2. \(\pi s\)

Acceleration of particle executing SHM at position is given as

⇒  \(|a| =\omega^2 y \) →  Equation 1

Here a = \(20 \mathrm{~ms}^{-1}\)

yy =\(5 \mathrm{~m}\)

20 =\(\omega^2(5)\)

⇒  \(\omega^2\) =4

⇒ \(\omega =2 \mathrm{rad} / \mathrm{s}\)

Time period, T =\(\frac{2 \pi}{\omega}=\frac{2 \pi}{2}=\pi \mathrm{s}\)

T =\(\pi s\)

Question 44. A spring of force constant k is cut into lengths of ratio 1: 2 : 3. They are connected in series and the new force constant is k. If they are connected in parallel and the force constant is k”, then K’: k” is:

1. 1: 6
2. 1: 9
3. 1: 11
4. 1: 14

Answer: 3. 1: 11

Spring constant \(\propto \frac{1}{\text { length }}\)

K=\(\frac{1}{l}\)

i.e. \(K_1=6 K, K_2=3 K and K_3\)=2 K

In series, \(\frac{1}{K^{\prime}}=\frac{1}{6 K}+\frac{1}{3 K}+\frac{1}{2 K}\)

⇒  \(\frac{1}{K^{\prime}}=\frac{6}{6 K}\)

⇒  \(K^{\prime}\)=K

⇒  \(K^{\prime \prime}=6 K+3 K+2 K=11 K\)

⇒ \(\frac{K^{\prime}}{K^{\prime \prime}}=\frac{1}{11}\)

∴ \(K^{\prime}: K^{\prime \prime}\)=1: 11

Question 45. A body of mass m is attached to the lower end of a spring whose end is fixed. The spring has negligible mass When the mass m is slightly pulled down and released, it oscillates with a time period of 3s. When the mass m is increased by 1 kg. The time period of oscillations becomes 5 s. The value of m in kg is:

1. \(\frac{3}{4}\)
2. \(\frac{4}{3}\)
3. \(\frac{16}{9}\)
4. \(\frac{9}{16}\)

Answer: 4. \(\frac{9}{16}\)

According to the question, the diagram is

Time period, T=\(2 \pi \sqrt{\frac{m}{k}}\)

⇒  \(\mathrm{I}^{\text {st }} \text { case, }=T_1=2 \pi \sqrt{\frac{m}{k}}\)  → Equation 1

⇒  \(II ^{\text {nd }}\) case when mass m is increased by 1 \(\mathrm{~kg}\) then mass becomes (m+1)

⇒  \(T_2=2 \pi \sqrt{\frac{m+1}{m}}\) →  Equation 2

from eq. (1) and (2) \(\omega_0\) get

⇒  \(\frac{T_2}{T_1}=\sqrt{\frac{m+1}{m}}\)

⇒  \(\frac{5}{3}=\sqrt{\frac{m+1}{m}}\)

⇒  \(\frac{m+1}{m}=\frac{25}{9} \)

⇒  \(1+\frac{1}{m}=\frac{25}{9}\)

∴ \( m=\frac{9}{16} \mathrm{~kg}\)

Question 46. The period of oscillation of a mass M suspended from a spring of negligible mass is T. If along with it another mass M is also suspended, the period of oscillation will now be:

1. T
2. \(\frac{T}{\sqrt{2}}\)
3. 2T
4. \(\sqrt{2} T\)

Answer: 4. \(\sqrt{2} T\)

Time period of simple pendulum T

-T = \(2 \pi \sqrt{\frac{\mathrm{M}}{k}}\)

When mass is double then \(T^{\prime} =2 \pi \sqrt{\frac{2 M}{k}}=\sqrt{2}\left(2 \pi \sqrt{\frac{M}{k}}\right)\)

= \(\sqrt{2} T\)

Question 47. A mass of 2.0 kg is put on a flat pan attached to a vertical spring fixed on the ground as shown in the figure. The mass of the spring and the pan is negligible. When pressed slightly and released the mass executes a simple harmonic motion. The spring constant is 200 N/m. What should be the minimum amplitude of the motion so that the mass gets detached from the pan (take g = 10 m/s²)?

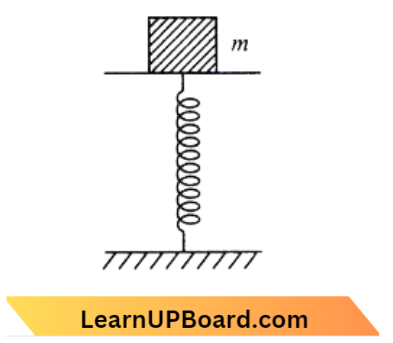
1. 10.0 cm
2. any value less than 12.0 cm
3. 4.0 cm
4. 8.0 cm

Answer: 1. 10.0 cm

For Given Condition, \(m g =m \omega^2 a=k a\)

a =\(\frac{m g}{k}=\frac{2 \times 10}{200}\)

=0.1=10 \(\mathrm{~cm}\)

Question 48. The time period of mass suspended from a spring is T. If the spring is cut into four equal parts and the same mass is suspended from one of the parts, then the new time period will be:

1. \(\frac{T}{4}\)
2. T
3. \(\frac{T}{2}\)
4. 2T

Answer: 3. \(\frac{T}{2}\)

Let K = force constant of spring and

K = force constant of each part then

⇒  \(\frac{1}{K} =\frac{4}{K^{\prime}}\)

⇒  \(K^{\prime}\) =4 K

Time period =\(2 \pi \sqrt{\frac{m}{4 K}}\)

= \(\frac{1}{2} \times 2 \pi \sqrt{\frac{m}{4 K}}=\frac{T}{2}\)

Question 49. The time period of a simple pendulum is 2 s. If its length is increased by 4 times, then its period becomes:

1. 16 s
2. 12 s
3. 8 s
4. 4 s

Answer: 4. 4 s

We have, time period of a simple pendulum

T=2 \(\pi \sqrt{\frac{l}{g}}\)

where, l= length of the pendulum

g= acceleration due to gravity

T \(\propto \sqrt{l}\)

Hence, \(\frac{T_2}{T_1}=\sqrt{\frac{l_2}{l_1}}\)  → Equation 1

Given, \(l_2=4 l_1, T_1=2 \mathrm{~s}\)

Substituting these values in Eq. (i), we get

⇒  \(T_2=\sqrt{\frac{4 l_1}{l_1}} \times 2=2 \times 2=4 \mathrm{~s}\)

Question 50. The damping force on an oscillator is directly proportional to the velocity. The units of the constant of proportionality are:

1. kg ms^-1
2. kg ms^-2
3. kg s^-1
4. kg s

Answer: 3. kg s^-1

It is given that force \(\propto\) velocity

⇒ \(F \propto V \)

F =K V

K = \(\frac{F}{V}\)

Unit of K =\(\frac{F}{V}=\mathrm{kg} \mathrm{s}^{-1}\)

Question 51. When an oscillator completes 100 oscillations its amplitude reduces to \(\frac{1}{3}\) of the initial value. What will be its amplitude when it completes 200 oscillations?

1. \(\frac{1}{8}\)
2. \(\frac{2}{3}\)
3. \(\frac{1}{6}\)
4. \(\frac{1}{9}\)

Answer: 1. \(\frac{1}{8}\)

Let \(a_0\) be the initial amplitude and b be the damping constant

The used formula is a=\(a_0 e^{-b t}\)

In Ist case : t=100 T and a=\(\frac{a_0}{3}\)

⇒ \(\frac{a_0}{3} =a_0 e^{-b(100 T)}\)

⇒ \(e^{-b \times 100 T} =\frac{1}{3}\)

In 2nd case : t =200 T

a = \( a_0 e^{-200 b T}\)

a = \(a_0\left(e^{-100 b T}\right)^2\)

a = \(a_0\left(\frac{1}{3}\right)^2\)

a = \(\frac{a_0}{9}\)

Question 52. In case of a forced vibration, the resonance peak becomes very sharp when the:

1. damping force is small
2. restoring force is small
3. applied periodic force is small
4. the quality factor is small

Answer: 1. The damping force is small

Less damping gives a taller and narrower resonance peak.